Introduction to Förster Resonance Energy Transfer (FRET)

In biological applications involving fluorescence microscopy, the application of Förster Resonance Energy Transfer (FRET) technique has increased considerably. This technique allows for molecular interactions and conformational changes. Through the Förster mechanism, fluorescence energy transfer occurs by means of a radiationless, coulombic, dipole-dipole interaction and remains active over the 10 to 100Å range. This makes the FRET technique suitable to probe nanoscale processes.

Resonance Energy Transfer

The coulombic, dipole-dipole interaction needs spectral overlap between an acceptor’s absorption and the donor emission, along with the appropriate orientation of their transition dipoles. This interaction is evident over the range of 10 to 100Å, making it suitable to track molecular interactions and ascertain distances on the nanometre scale. This fact is leveraged in microscopy to examine interactions beyond the optical diffraction limit. Figure 1 shows the possibility of non radiative resonance coupling from donor (D) to acceptor (A) as an optional means for the donor to return to the ground state.

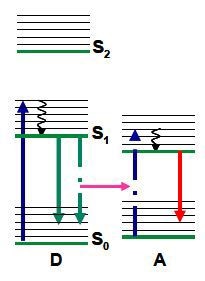
Figure 1. Scheme for resonance energy transfer, shown using a Jablonski diagram.

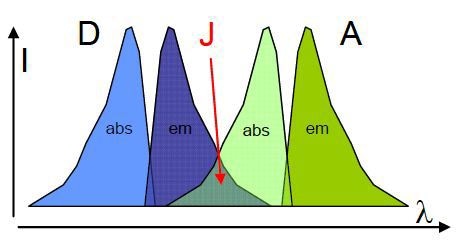
Figure 2. Representation of the spectral overlap (J) of a donor (D) emission and acceptor (A) absorption.

Figure 2 shows the strength of the spectral overlap, which is distinguished by the overlap integral (J); J can be shown as,

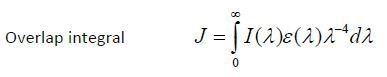
This contributes to the critical transfer distance, which is also called as the Förster distance (R₀); critical transfer distance is the distance at which energy transfer is 50% efficient. The formula is shown below:

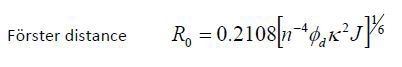
Where n stands for the refractive index, Φ_d is the donor fluorescence quantum yield, and κ is the orientation factor for the transition dipoles. For a random orientation κ² is 2/3, which is the distance at which the possibility of the excited state donor emitting fluorescence or transferring energy is 50%.

The rate of energy transfer (k_et) can be shown as,

τ_d represents the fluorescence lifetime of the donor, without the presence of an acceptor. The equation shown above demonstrates that there is a R⁶ distance dependence on the rate of energy transfer, which influence the lifetime of the donor in the presence of the acceptor (τ_da). This R⁶ dependency gives the use of FRET its sensitivity. When the donor lifetime is measured, it allows both the efficiency (E) of energy transfer and the distance (R) to be measured. Only when the lifetime of the unquenched donor τ_d is known, the Förster distance (R₀) is also known for the specific system being studied.

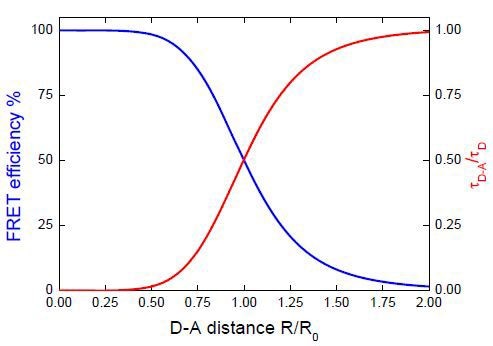
Figure 3. Influence of donor-acceptor distance on the donor lifetime and FRET efficiency.

Figure 3 shows the effect of the donor-acceptor distance on the efficiency of energy transfer and the donor lifetime. This demonstrates that the most sensitive distance region is 0.5 to 1.5 times the R₀ value.

Applications of FRET

Recommended FRET pairs are often there for particular applications. They may be free or tethered in solution. In the former case, the solvent must have high viscosity to shorten the molecular diffusion length with respect to R₀. This method needs more number of acceptors and the information can then be fitted to a form of stretched exponential. Diffusion can be included through Yokota-Tanimoto fitting by making certain adjustments.

For a FRET pair, the optimum R₀ is between 40 to 60Å so as to leverage its ability to sense nanoscale distances. Nevertheless, distance information may be exposed, as the direction of the transition dipoles have to be accounted for. Hence, analysis of the study results should also take into account whether the relative orientation and the distance of the acceptor and donor also changes.

Conclusion

FRET has been used in a number of applications. A few examples include characterization of microheterogenous, photosynthetic systems, polymers, protein interactions, protein conformation, media, and biosensors. To sum up, the main usage of FRET is where there is a need to determine changes in distance on the nanoscale, either by two molecules approaching and interacting or within a macromolecule.

This information has been sourced, reviewed and adapted from materials provided by HORIBA.

For more information on this source, please visit HORIBA.