Jan 9 2019
Recently, three scientists from the University of Massachusetts Amherst, addressing a physics problem that dates back to Galileo, have suggested a new method to the theory of how thin sheets can be forced to conform to “geometrically incompatible” shapes—similar to gift-wrapping a basketball—that depends on intertwining two fundamental ideas of geometry and mechanics that were long believed to be incompatible.
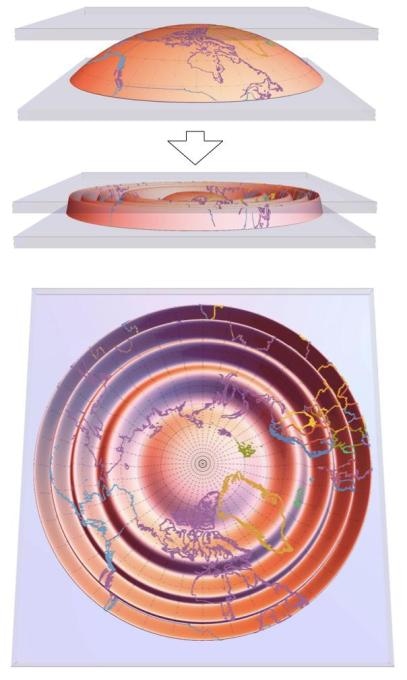Schematic shows what happens when a curved elastic shell is forced by confinement to change from spherical to flat. (Photo courtesy UMass Amherst/G. Grason)
Theoretical physicist Benny Davidovitch, polymer scientist Greg Grason and doctoral student Yiwei Sun, writing in Proceedings of the National Academy of Sciences, propose and demonstrate via numerical simulations that naturally flat sheets forced to alter their curvature can house geometrically-required strain by forming microscopic wrinkles that bend the sheet rather than stretching it to the breaking point, a solution that consumes less energy, as well.
This progress is crucial as biotechnologists increasingly try to manipulate the level of strain encountered in thin films compliant to complex, curving and 3D shapes of the human body, for instance, in flexible and wearable sensors for personalized health monitoring, they describe. A number of these devices depend on the electrical properties of the film which is shown to be extremely susceptible to stretching, but which can tolerate a certain amount of bending.
The new concept is one of “close enough,” Davidovitch says—nonconformities that come with bending are so small that in practical scenarios, they cost virtually no energy. “By offering efficient strategies to manage the strain, predict it and control it, we offer a new quantitative tool that’s useful for people predicting the forces required to emboss or wrap nanoscopic thin sheets and shells onto substrates of different shapes,” they state.
He adds, “Our work shows that by allowing tiny wrinkles in the wrapper, the necessary amount of stretching drops dramatically. For an extremely thin wrapper such as available today in laboratories, the stretching can be eliminated almost entirely.”
Our theoretical framework provides a simple and adaptable tool for understanding how to control and manipulate, and ideally to optimize, the level of strain that a given geometry imposes on such a device, and thereby improve its performance.
Greg Grason, Polymer Scientist, University of Massachusetts Amherst.
Davidovitch says there are two types of researchers fascinated in this long-standing problem, one less driven by practicality than by how the laws of Nature apply. These thinkers are acquainted with “Galileo’s beam,” a mechanics/physics problem that imagined a beam sticking out of a stone wall that will deform or bend when weight is added to it, he describes. Predicting the forces and strain on it posed an age-old puzzle.
Galileo did not solve how much the beam will deform or how to foretell that, he observes, but this problem associated with strain was later investigated and defined through new approaches to the geometry of continuous objects by German mathematician and physicist Carl Friedrich Gauss. Physicists and mathematicians “have focused a lot of intellectual activity over the centuries on it,” Davidovitch says.
After Galileo, Davidovitch says, Swiss mathematician Leonhard Euler developed the “elastica theory,” which claims that confined objects buckle so as to avoid strain, that is, any alteration in length. Euler demonstrated that a situation in which totally no stretching takes place can occur under special conditions, but not in the general type of confinement demarcated by Gauss’s geometrical constraints, he adds.
The UMass Amherst team’s new tool demonstrates—when a constraint cannot be seamlessly satisfied but nearly satisfied—how to find the physical shape or state that is the best fit. “It’s a new branch of variational calculus,” Davidovitch says. “All I need to do is minimize the curvature that nearly eliminates all stretching, and it lets me find the one with the smallest possible bending energy.”
They suggest a new principle, the Gauss-Euler elastica that reconciles the two keystones of classical mechanics and geometry defined earlier by the works of Euler and Gauss. They invoke a new regime of solutions of the multifaceted morphologies of thin bodies, an issue of intense recent interest from materials engineering and biophysics to applied mathematics, Grason highlights.
He recalls, “When we first started this line of research, we didn’t imagine how it would turn out. We were not trying to solve this problem.” But doctoral student Sun, running a few computer simulations, delivered results that challenged naïve assumptions that confinement requires more energy to stretch than to bend the sheet. Formulas that he and Grason projected were “impossible,” Davidovitch says, “they appeared to be violating fundamental geometrical theorems.”
They were unsuccessful for two years, until they recalled Galileo’s beam, what Davidovitch terms as “thought contradiction,” that re-framed the question. It has been “very satisfying” to offer a new conceptual tool to the problem that did not exist before, he says.
It’s great to have an answer to why our initial simulations behaved so weirdly, of course. But eventually, it leads us to understand the question better, and how it addresses a much broader class of problems in a new way. Yes, this has a good feeling.
Greg Grason, Polymer Scientist, University of Massachusetts Amherst.